How to decouple noise and sharp features on complex geometric construction? This question still lies across researchers’ studying now. Detecting noises and sharp features on 3D shapes is regarded as a challenge worldwide, because many geometry processing applications are sensitive to both noises and sharp shapes. Traditional methods of filtering them are not reliable and robust, but in April, 2014, a new approach for decoupling them was proposed by Graphics&Geometric Computing Laboratory at USTC.

Different from traditional ways, this group adopts two-phase methods to denoise the input mesh and to recover the feature. To prove their theory, they have tested this approach a large number of mesh surfaces with various feature types. Experimental results have shown that this method can faithfully recover 3D features corrupted by different levels of noise, even by heavily added noise.

Here shows some interesting experimental results (ACM Transactions on Graphics, 33(2). Article 18:1-12, March 2014. DOI: http://dx.doi.org/10.1145/2557449).A severe noise signal was added to the 8-shaped surface model. Their algorithm can automatically find the optimal value of λ via GCV method.

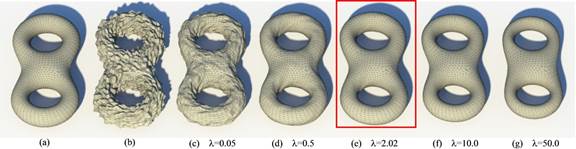
Figure1. Denoising the –shaped mesh: (a) the ground-truth; (b) the model corrupted by severe noise; (c)–(g) denoised results by the global smoothing approach with various parameters λ. (e) λ=2.02 is the best one.

To validate accuracy of this algorithm, they even build quite complex models with many sharp features, like cube model, octa-flower model, and all of them are correctly recovered with slight difference with the ground truth.

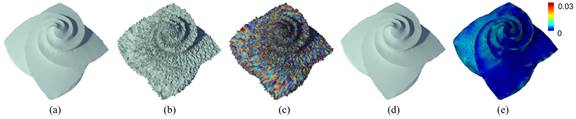
Figure 2. An example of recovering sharp shapes corruped by noise: (a) The octa-flower model as a ground truth; (b) the model corrupted by independent and identically distributed (i.i.d.) noise; (c) the error map of (b); (d) the denoising result by their approach; (e) the error map of (d).

This breakthrough was highly appraised by the reviewers of ACM Transactions on Graphics and the paper was published in this top journal recently. The first author of this paper is WANG Ruimin and the corresponding author is YANG Zhouwang, an associate professor in USTC. In Aug, 2014, the author will give a speech in Special Interest Group for Computer Graphics (SIGGRAPH) Conference in Canada.

(CHEN Yaoyao, USTC News Center)